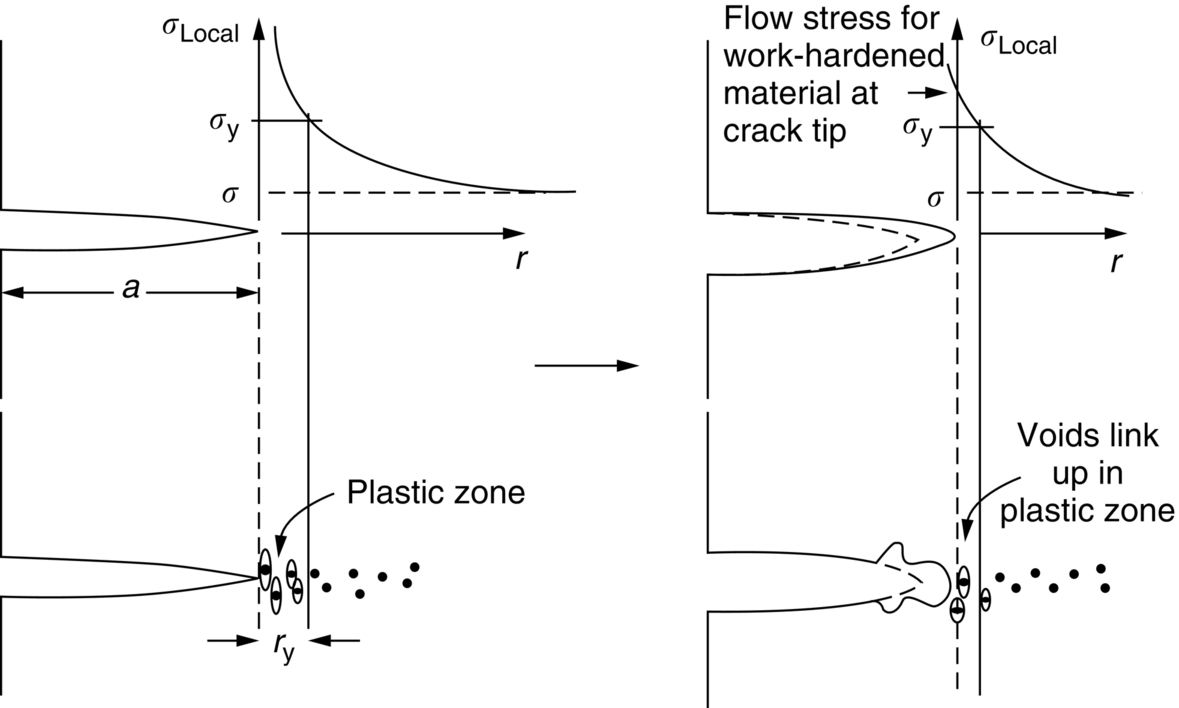
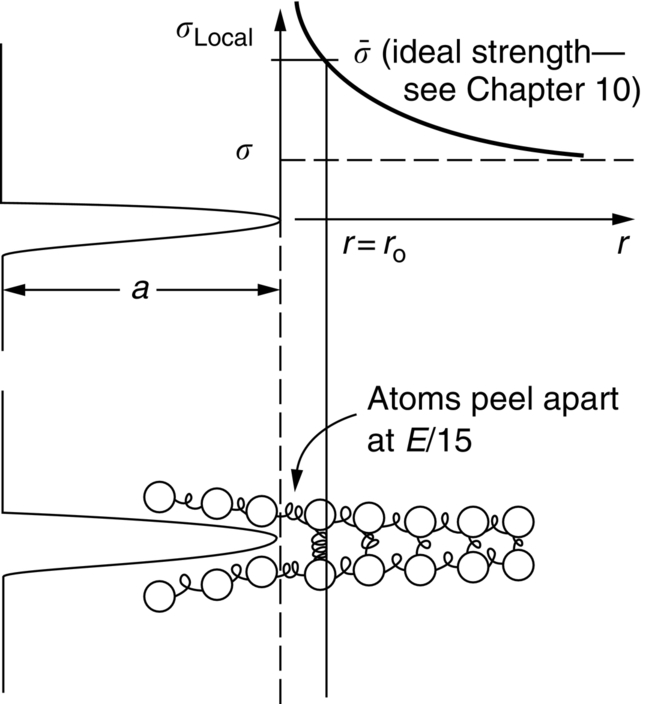
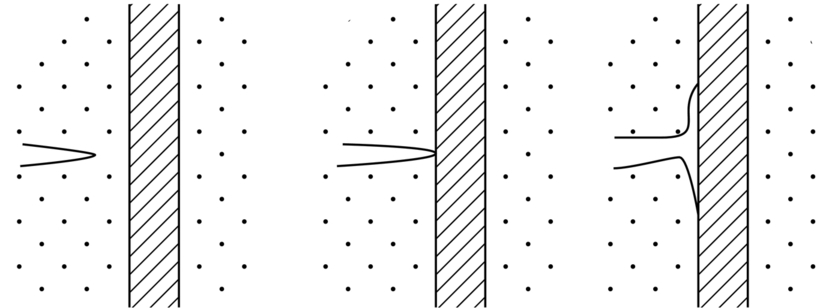
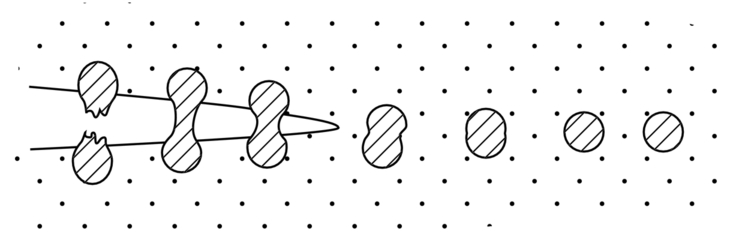
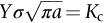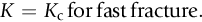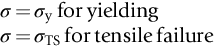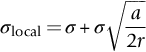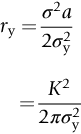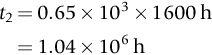section epub:type=”chapter”> This chapter focuses on micromechanisms of fast fracture. The chapter discusses the mechanisms of crack propagation such as ductile tearing and cleavage. The chapter first takes a look at what happens when a cracked piece of a ductile metal is loaded —in other words, a metal that can flow readily to give large plastic deformations (e.g., pure copper; or mild steel at, or above, room temperature). If the material is sufficiently loaded, it can start to get fractured from the crack. If one examines the surfaces of the metal after it has fractured he will see that the fracture surface is extremely rough, indicating that a great deal of plastic work has taken place. Whenever a crack is present in a material, the stress close to the crack, σlocal, is greater than the average stress σ applied to the piece of material; the crack has the effect of concentrating the stress. The next section of this chapter discusses about composites, including wood. The low toughness of materials, such as epoxy resins or polyester resins, can be enormously increased by reinforcing them with carbon fiber or glass fiber. But why is it that putting a second, equally (or more) brittle material such as graphite or glass into a brittle polymer makes a tough composite? The reason is the fibers act as crack stoppers. This chapter concludes with a discussion of the way to avoid brittle alloys. In Chapter 14 we showed that, if a material contains a crack and is sufficiently stressed, the crack becomes unstable and grows—at up to the speed of sound in the material—to cause catastrophically rapid fracture, or fast fracture at a stress less than the yield stress. We were able to quantify this phenomenon and obtained a relationship for the onset of fast fracture or, in shorter notation, It is helpful to compare this with other, similar, failure criteria: The left side of each equation describes the loading conditions; the right side is a material property. When the left side (which increases with load) equals the right side (which is fixed), failure occurs. Some materials, such as glass, have low Kc, and crack easily; ductile metals have high Kc and are very resistant to fast-fracture; polymers have intermediate Kc, but can be made tougher by making them into composites; and (finally) many metals, when cold, become brittle—that is, Kc decreases with temperature. How can we explain these important observations? Let us first of all look at what happens when we load a cracked piece of a ductile metal—in other words, a metal that can flow readily to give large plastic deformations (e.g., pure copper; or mild steel at, or above, room temperature). If we load the material sufficiently, we can get fracture to take place starting from the crack. If you examine the surfaces of the metal after it has fractured (Figure 15.1) you see that the fracture surface is extremely rough, indicating that a great deal of plastic work has taken place. Let us explain this observation. Whenever a crack is present in a material, the stress close to the crack, σlocal, is greater than the average stress σ applied to the piece of material; the crack has the effect of concentrating the stress. Mathematical analysis shows that the local stress ahead of a sharp crack in an elastic material is The closer one approaches to the tip of the crack, the higher the local stress becomes, until at some distance ry from the tip of the crack the stress reaches the yield stress, σy of the material, and plastic flow occurs (Figure 15.2). The distance ry is easily calculated by setting σlocal = σy in Equation (15.1). Assuming ry to be small compared to the crack length, a, the result is The crack propagates when K is equal to Kc; the width of the plastic zone, ry, is then given by Equation (15.2) with K replaced by Kc. Note that the zone of plasticity shrinks rapidly as σy increases: cracks in soft metals have a large plastic zone; cracks in hard ceramics have a small zone, or none at all. Even when nominally pure, most metals contain tiny inclusions (or particles) of chemical compounds formed by reaction between the metal and impurity atoms. Within the plastic zone, plastic flow takes place around these inclusions, leading to elongated cavities, as shown in Figure 15.2. As plastic flow progresses, these cavities link up, and the crack advances by means of this ductile tearing. The plastic flow at the crack tip naturally turns our initially sharp crack into a blunt crack, and it turns out from the stress mathematics that this crack blunting decreases σlocal so that, at the crack tip itself, σlocal is just sufficient to keep on plastically deforming the work-hardened material there, as the figure shows. The important thing about crack growth by ductile tearing is that it consumes a lot of energy by plastic flow; the bigger the plastic zone, the more energy is absorbed. High energy absorption means that Gc is high, and so is Kc. This is why ductile metals are so tough. Other materials, too, owe their toughness to this behavior—plasticine is one, and some polymers also exhibit toughening by processes similar to ductile tearing. If you now examine the fracture surface of something like a ceramic, or a glass, you see a very different situation. Instead of a very rough surface, indicating massive local plastic deformation, you see rather flat surfaces, suggesting little or no plastic deformation. How is it that cracks in ceramics or glasses can spread without plastic flow taking place? Well, the local stress ahead of the crack tip, given by our formula
Micromechanisms of Fast Fracture
Publisher Summary
15.1 Introduction
15.2 Mechanisms of Crack Propagation 1: Ductile Tearing
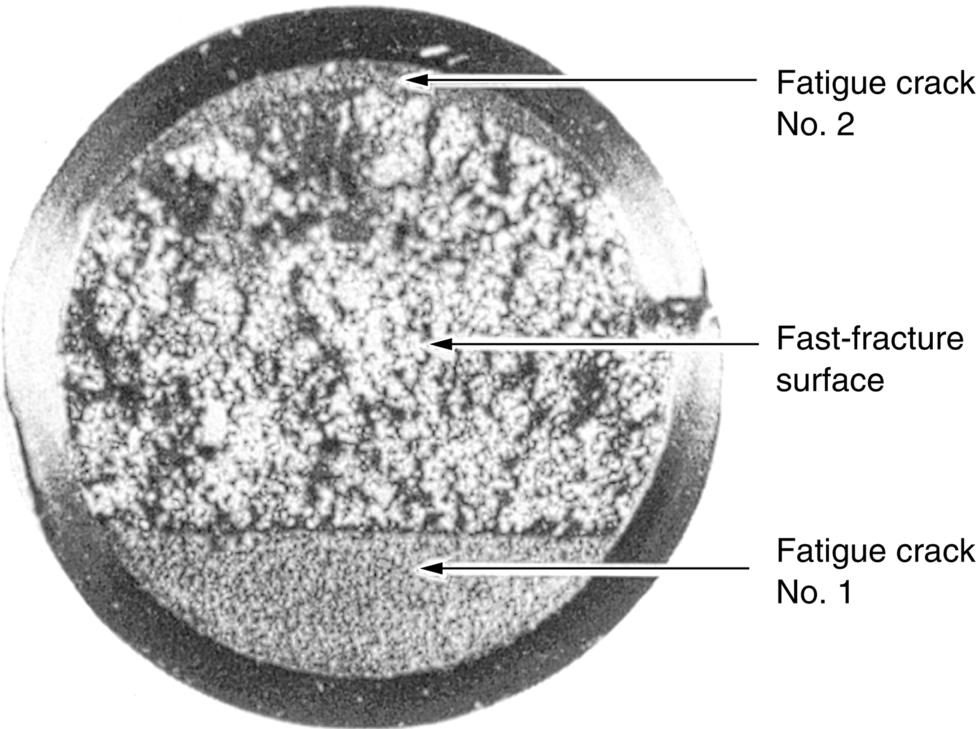
15.3 Mechanisms of Crack Propagation 2: Cleavage
